Next: 2.7 Discussion and Exercises Up: 2. Array-Based Lists Previous: 2.5 DualArrayDeque: Building a Contents Index
One of the drawbacks of all previous data structures in this chapter is
that, because they store their data in one or two arrays and they avoid
resizing these arrays too often, the arrays frequently are not very full.
For example, immediately after a
![]() operation on an ArrayStack,
the backing array
operation on an ArrayStack,
the backing array
![]() is only half full. Even worse, there are times
when only one third of
is only half full. Even worse, there are times
when only one third of
![]() contains data.
contains data.
In this section, we discuss the RootishArrayStack data structure,
that addresses the problem of wasted space. The RootishArrayStack
stores
![]() elements using
elements using
![]() arrays. In these arrays, at
most
arrays. In these arrays, at
most
![]() array locations are unused at any time. All
remaining array locations are used to store data. Therefore, these
data structures waste at most
array locations are unused at any time. All
remaining array locations are used to store data. Therefore, these
data structures waste at most
![]() space when storing
space when storing
![]() elements.
elements.
A RootishArrayStack stores its elements in a list of
![]() arrays called blocks that are numbered
arrays called blocks that are numbered
![]() .
See Figure 2.5. Block
.
See Figure 2.5. Block ![]() contains
contains ![]() elements.
Therefore, all
elements.
Therefore, all
![]() blocks contain a total of
blocks contain a total of
![\includegraphics[width=\textwidth ]{figs-python/rootisharraystack}](img930.png)
|
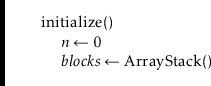
![\includegraphics[scale=0.90909]{figs-python/gauss}](img934.png)
|
As we might expect, the elements of the list are laid out in order
within the blocks. The list element with index 0 is stored in block 0,
elements with list indices 1 and 2 are stored in block 1, elements with
list indices 3, 4, and 5 are stored in block 2, and so on. The main
problem we have to address is that of determining, given an index
![]() ,
which block contains
,
which block contains
![]() as well as the index corresponding to
as well as the index corresponding to
![]() within that block.
within that block.
Determining the index of
![]() within its block turns out to be easy. If
index
within its block turns out to be easy. If
index
![]() is in block
is in block
![]() , then the number of elements in blocks
, then the number of elements in blocks
![]() is
is
![]() . Therefore,
. Therefore,
![]() is stored at location
is stored at location
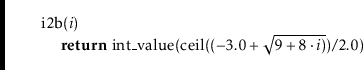
With this out of the way, the
![]() and
and
![]() methods are straightforward. We first compute the appropriate block
methods are straightforward. We first compute the appropriate block
![]() and the appropriate index
and the appropriate index
![]() within the block and then perform the appropriate operation:
within the block and then perform the appropriate operation:

If we use any of the data structures in this chapter for representing the
![]() list, then
list, then
![]() and
and
![]() will each run in constant time.
will each run in constant time.
The
![]() method will, by now, look familiar. We first check
to see if our data structure is full, by checking if the number of
blocks,
method will, by now, look familiar. We first check
to see if our data structure is full, by checking if the number of
blocks,
![]() , is such that
, is such that
![]() . If so, we call
. If so, we call
![]() to add another block. With this done, we shift elements with indices
to add another block. With this done, we shift elements with indices
![]() to the right by one position to make room for the
new element with index
to the right by one position to make room for the
new element with index
![]() :
:

The
![]() method does what we expect. It adds a new block:
method does what we expect. It adds a new block:
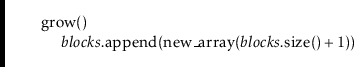
Ignoring the cost of the
![]() operation, the cost of an
operation, the cost of an
![]() operation is dominated by the cost of shifting and is therefore
operation is dominated by the cost of shifting and is therefore
![]() , just like an ArrayStack.
, just like an ArrayStack.
The
![]() operation is similar to
operation is similar to
![]() . It shifts the
elements with indices
. It shifts the
elements with indices
![]() left by one position and then,
if there is more than one empty block, it calls the
left by one position and then,
if there is more than one empty block, it calls the
![]() method
to remove all but one of the unused blocks:
method
to remove all but one of the unused blocks:


Once again, ignoring the cost of the
![]() operation, the cost of
a
operation, the cost of
a
![]() operation is dominated by the cost of shifting and is
therefore
operation is dominated by the cost of shifting and is
therefore
![]() .
.
The above analysis of
![]() and
and
![]() does not account for
the cost of
does not account for
the cost of
![]() and
and
![]() . Note that, unlike the
. Note that, unlike the
![]() operation,
operation,
![]() and
and
![]() do not copy
any data. They only allocate or free an array of size
do not copy
any data. They only allocate or free an array of size
![]() . In
some environments, this takes only constant time, while in others, it
may require time proportional to
. In
some environments, this takes only constant time, while in others, it
may require time proportional to
![]() .
.
We note that, immediately after a call to
![]() or
or
![]() , the
situation is clear. The final block is completely empty, and all other
blocks are completely full. Another call to
, the
situation is clear. The final block is completely empty, and all other
blocks are completely full. Another call to
![]() or
or
![]() will
not happen until at least
will
not happen until at least
![]() elements have been added or removed.
Therefore, even if
elements have been added or removed.
Therefore, even if
![]() and
and
![]() take
take
![]() time, this
cost can be amortized over at least
time, this
cost can be amortized over at least
![]()
![]() or
or
![]() operations, so that the amortized cost of
operations, so that the amortized cost of
![]() and
and
![]() is
is
![]() per operation.
per operation.
Next, we analyze the amount of extra space used by a RootishArrayStack. In particular, we want to count any space used by a RootishArrayStack that is not an array element currently used to hold a list element. We call all such space wasted space.
The
![]() operation ensures that a RootishArrayStack never has
more than two blocks that are not completely full. The number of blocks,
operation ensures that a RootishArrayStack never has
more than two blocks that are not completely full. The number of blocks,
![]() , used by a RootishArrayStack that stores
, used by a RootishArrayStack that stores
![]() elements therefore
satisfies
elements therefore
satisfies
Next, we argue that this space usage is optimal for any data structure
that starts out empty and can support the addition of one item at
a time. More precisely, we will show that, at some point during the
addition of
![]() items, the data structure is wasting an amount of space
at least in
items, the data structure is wasting an amount of space
at least in
![]() (though it may be only wasted for a moment).
(though it may be only wasted for a moment).
Suppose we start with an empty data structure and we add
![]() items one
at a time. At the end of this process, all
items one
at a time. At the end of this process, all
![]() items are stored in
the structure and distributed among a collection of
items are stored in
the structure and distributed among a collection of
![]() memory blocks.
If
memory blocks.
If
![]() , then the data structure must be using
, then the data structure must be using
![]() pointers (or references) to keep track of these
pointers (or references) to keep track of these
![]() blocks, and these
pointers are wasted space. On the other hand, if
blocks, and these
pointers are wasted space. On the other hand, if
![]() then, by the pigeonhole principle, some block must have a size of at
least
then, by the pigeonhole principle, some block must have a size of at
least
![]() . Consider the moment at which this block
was first allocated. Immediately after it was allocated, this block
was empty, and was therefore wasting
. Consider the moment at which this block
was first allocated. Immediately after it was allocated, this block
was empty, and was therefore wasting
![]() space. Therefore,
at some point in time during the insertion of
space. Therefore,
at some point in time during the insertion of
![]() elements, the data
structure was wasting
elements, the data
structure was wasting
![]() space.
space.
The following theorem summarizes our discussion of the RootishArrayStack data structure:
The space (measured in words)2.2 used by a RootishArrayStack
that stores
![]() elements is
elements is
![]() .
.